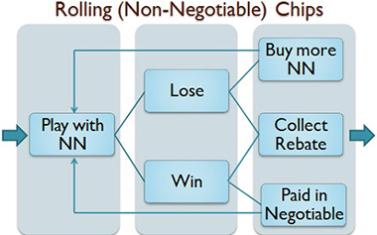
Macau has a long-standing tradition of rewarding the high roller with a rebate, according to his original buy-in and total volume of play. This all takes place in private VIP casinos, and often through an entity between the player and the casino, called a junket. Some casinos will offer such programs directly to the player, as discussed in more detail later.
If going through a junket, the junket operator will offer credit, dictate the Dead Chip terms to the players, and participate with the casino on some basis in the overall win or loss. These junket operators cater to mainland Chinese players. When I went to Macau I was harshly rebuked in Cantonese for getting anywhere close to these VIP rooms. It is my understanding, based on talking with Macau clients, that junket operators do not like to advertise, nor deal with the general public. Instead they prefer to do business on an honor, and word of mouth basis, in a limited area in China that the junket operator knows.
Here is how it works. Whenever the player buys chips, he will be given Dead Chips, or what we would call in the U.S. "non-negotiable" or "free play" chips. These chips the player holds until lost. If the player wins a bet, he keeps the Dead Chips, and is paid in cashable chips. Nothing happens with a push, and the Dead Chip is lost if the bet loses. The only game Dead Chips may be wagered on is baccarat.
Eventually, the player will work through all his Dead Chips, and unless he lost every bet, will be left with cashable chips. He can then use the cashable chips to repurchase more Dead Chips. This is conveniently done with the aid of pretty girls who run the chips back and forth between the player and the cashier. With every such transaction, the cashier will note the total Dead Chips purchased.
After the player has worked through his last chip rotation, he will ask for his rebate. The cashier will give him chips (usually cashable ones) equal to the product of the agreed-upon rebate percentage and total Dead Chips purchased.
Let's look at an example. Suppose the player has a credit line of $50,000 and is offered a 0.5% Dead Chip rebate. He purchases 50 $1,000 Dead Chips. After working through all of them, he runs his balance up to $55,000 in cashable chips. Then he turns those cashable chips back into $55,000 in Dead Chips. After the second rotation he brings his balance down to $48,000 in cashable chips. He then does a third rotation, turning the $48,000 in Dead Chips to $44,000 in cashable chips. At this point he asks for his rebate, which would be 0.005 × ($50,000 + $55,000 + $48,000) = $765. His final balance is $44,765.
I thought for a while about how to quantify the value of these Dead Chip programs. The expected value of a dead chip is as follows.
1 - h × n =
1 - h / p
Where:
h = house edge,
n = the number of bets expected until the Dead Chip is lost,
p = probability of losing
In the case of the Banker bet, that is 1 — 1.0579% / 0.446247 = 97.63% of face value. Even with a 1% rebate, that would still make the value less than a single cashable wager on the Banker bet. Does that mean the player should refuse a Dead Chip program? No, of course not. What I think is appropriate to measure is the ratio of the expected loss to the total amount of action, which is significantly reduced with a Dead Chip program.
The first table shows the financial value of the Dead Chips, according to the rebate and the bet made. Another way to express it is the return on investment of each Dead Chip purchase. This table is of little value to the gambler, but would be of interest to someone who took no pleasure in gambling.
| Value of Dead Chips to the Non-Gambler | ||||
|---|---|---|---|---|
| Rebate | Banker | Player | Tie | Pair |
| 0% | 97.629% | 97.307% | 84.13% | 88.802% |
| 0.1% | 97.727% | 97.404% | 84.214% | 88.891% |
| 0.2% | 97.825% | 97.501% | 84.299% | 88.98% |
| 0.3% | 97.922% | 97.599% | 84.383% | 89.068% |
| 0.4% | 98.02% | 97.696% | 84.467% | 89.157% |
| 0.5% | 98.117% | 97.793% | 84.551% | 89.246% |
| 0.6% | 98.215% | 97.891% | 84.635% | 89.335% |
| 0.7% | 98.313% | 97.988% | 84.719% | 89.424% |
| 0.8% | 98.41% | 98.085% | 84.803% | 89.513% |
| 0.9% | 98.508% | 98.183% | 84.887% | 89.601% |
| 1.0% | 98.606% | 98.28% | 84.972% | 89.69% |
| 1.5% | 99.094% | 98.766% | 85.392% | 90.134% |
The next table shows the expected loss to the total action. This, I believe, is the appropriate measure of the house edge, after adjusting for the Dead Chip program.
| Adjusted House Edge after Applying Dead Chip Rebate | ||||
|---|---|---|---|---|
| Rebate | Banker | Player | Tie | Pair |
| 0% | 1.058% | 1.235% | 14.36% | 10.361% |
| 0.1% | 1.014% | 1.190% | 14.284% | 10.279% |
| 0.2% | 0.971% | 1.146% | 14.207% | 10.197% |
| 0.3% | 0.927% | 1.101% | 14.131% | 10.115% |
| 0.4% | 0.884% | 1.057% | 14.055% | 10.033% |
| 0.5% | 0.840% | 1.012% | 13.979% | 9.951% |
| 0.6% | 0.797% | 0.967% | 13.903% | 9.868% |
| 0.7% | 0.753% | 0.923% | 13.827% | 9.786% |
| 0.8% | 0.709% | 0.878% | 13.751% | 9.704% |
| 0.9% | 0.666% | 0.833% | 13.675% | 9.622% |
| 1.0% | 0.622% | 0.789% | 13.598% | 9.540% |
| 1.5% | 0.404% | 0.566% | 13.218% | 9.129% |
The next table shows the percentage by which the Dead Chip program reduces the player's expected loss. For example, an 0.5% rebate applied to a player who only bet the banker, would lower his expected loss by 20.591%.
| Reduction in House Edge | ||||
|---|---|---|---|---|
| Rebate | Banker | Player | Tie | Pair |
| 0% | 0% | 0% | 0% | 0% |
| 0.1% | 4.118% | 3.613% | 0.530% | 0.793% |
| 0.2% | 8.236% | 7.226% | 1.060% | 1.586% |
| 0.3% | 12.355% | 10.839% | 1.590% | 2.379% |
| 0.4% | 16.473% | 14.452% | 2.121% | 3.172% |
| 0.5% | 20.591% | 18.065% | 2.651% | 3.965% |
| 0.6% | 24.709% | 21.679% | 3.181% | 4.758% |
| 0.7% | 28.827% | 25.292% | 3.711% | 5.551% |
| 0.8% | 32.946% | 28.905% | 4.241% | 6.344% |
| 0.9% | 37.064% | 32.518% | 4.771% | 7.137% |
| 1.0% | 41.182% | 36.131% | 5.301% | 7.930% |
| 1.5% | 61.773% | 54.196% | 7.952% | 11.895% |
The last set of tables show the Dead Chip rebate percentages that I am aware of. These are available when dealing directly with the casino, as opposed to going through a junket. The bankroll amounts are the minimum required for the given percentages. The comp rebate may be used for room, food, and beverages purchased in the casino/hotel. All bankroll amounts are in Hong Kong dollars.
| Dead Chip Rebates — Lisboa | |||
|---|---|---|---|
| Bankroll | Cash | Comps | Total |
| HK $ 200,000 | 0.4% | 0.1% | 0.5% |
| HK $ 300,000 | 0.5% | 0.1% | 0.6% |
| HK $ 1,000,000 | 0.6% | 0.1% | 0.7% |
| HK $ 5,000,000 | 0.7% | 0.1% | 0.8% |
| Dead Chip Rebates — Sands/Venetian | |||
|---|---|---|---|
| Bankroll | Cash | Comps | Total |
| HK $ 300,000 | 0.5% | 0.1% | 0.6% |
| HK $ 1,000,000 | 0.6% | 0.2% | 0.8% |
| HK $ 8,000,000 | 0.7% | 0.2% | 0.9% |
| Dead Chip Rebates — Starworld | |||
|---|---|---|---|
| Bankroll | Cash | Comps | Total |
| HK $ 300,000 | 0.5% | 0.1% | 0.6% |
| Dead Chip Rebates — Wynn | |||
|---|---|---|---|
| Bankroll | Cash | Comps | Total |
| HK $ 300,000 | 0.5% | 0.1% | 0.6% |
| HK $ 1,000,000 | 0.6% | 0.2% | 0.8% |
| HK $ 5,000,000 | 0.7% | 0.2% | 0.9% |
| Dead Chip Rebates — Foxwoods (Connecticut) | |||
|---|---|---|---|
| Bankroll | Cash | Comps | Total |
| US $ ? | 1.5% | 0% | 1.5% |
I would be interested to hear directly from any other Macau casinos that offer Dead Chip programs directly to the public.
Nov 12 2015



Comments
No comments for this article.